295
you are viewing a single comment's thread
view the rest of the comments
view the rest of the comments
this post was submitted on 14 Sep 2024
295 points (85.4% liked)
Math Memes
1156 readers
1 users here now
Memes related to mathematics.
Rules:
1: Memes must be related to mathematics in some way.
2: No bigotry of any kind.
founded 2 years ago
MODERATORS
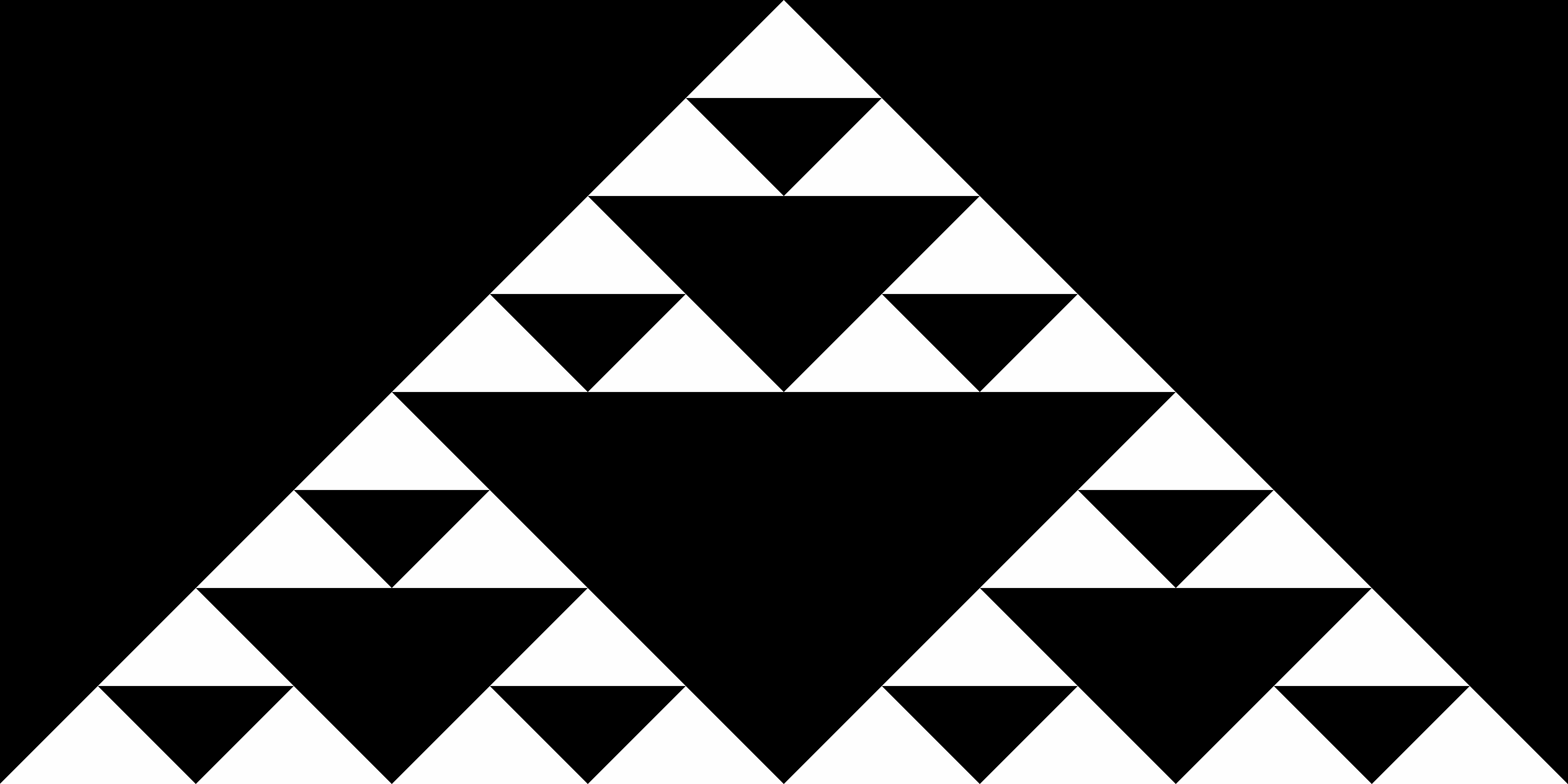
All possible formulas for the amount of triangles are as follows.
Let A(n) be the total number white of triangles in the n’th run.
Let B(n) be the total number of triangles in the n’th run in the structure that does not include the two corner triangles.
Let C(n) be the total numbers of triangles in the n’th run including the corner triangles.
Then we the following starting numbers and recursive formulas hold:
A(1)=1 and A(n+1)=3A(n) for all integers n>0
Reason: previous structure is embedded exactly 3 times
B(1)=1 and B(n+1)=3B(n) + 2 for all integers n>0
Reason: previous structure is embedded 3 times and this creates 1 new center triangle and 1 new greater triangle
C(1)=3 and C(n+1)=3(C(n) - 2) + 4 for all integers n>0
Reason: previous structure with the corner triangles removed is embedded 3 times, similar to previous case, and we add the 2 corner triangles at the end.
For this run (n=4) that means A(4)=27 B(4)=53 C(3)=55